Breadcrumb
Parameterized test patterns methodology for layout design rule checking verification
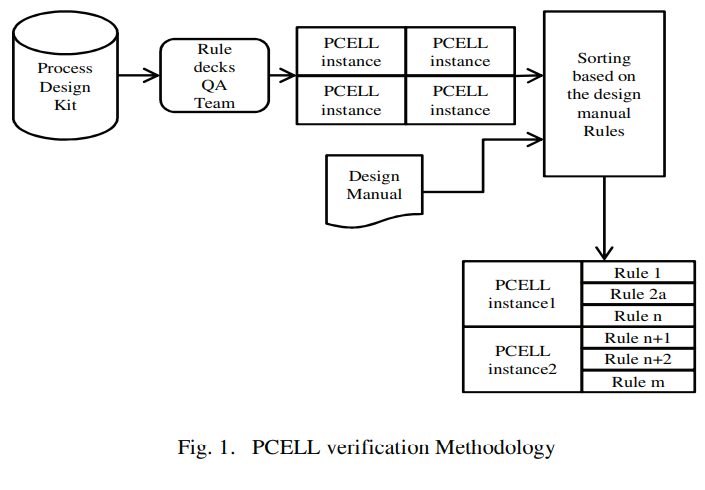
Design rules verification is an essential stage in the Process Design Kit (PDK) release for any fab. Since achieving high yield is the target of any fab, the design rules should ensure this. Design rules violations happening after fabrication lead to disastrous results on the mask sets as well as increased cost and delayed schedules. Here comes the importance of verifying these design rules and making sure that they represent the process in a manner that achieves a high yield and detects design rules issues early on. The verification process consumes 60% of the release cycle and the most time
Generalized smooth transition map between tent and logistic maps
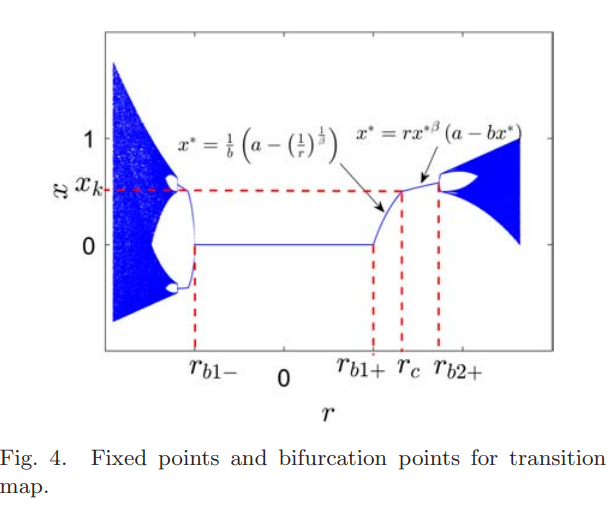
There is a continuous demand on novel chaotic generators to be employed in various modeling and pseudo-random number generation applications. This paper proposes a new chaotic map which is a general form for one-dimensional discrete-time maps employing the power function with the tent and logistic maps as special cases. The proposed map uses extra parameters to provide responses that fit multiple applications for which conventional maps were not enough. The proposed generalization covers also maps whose iterative relations are not based on polynomials, i.e. with fractional powers. We introduce
An eight-term 3-D novel chaotic system with three quadratic nonlinearities, its adaptive feedback control and synchronization
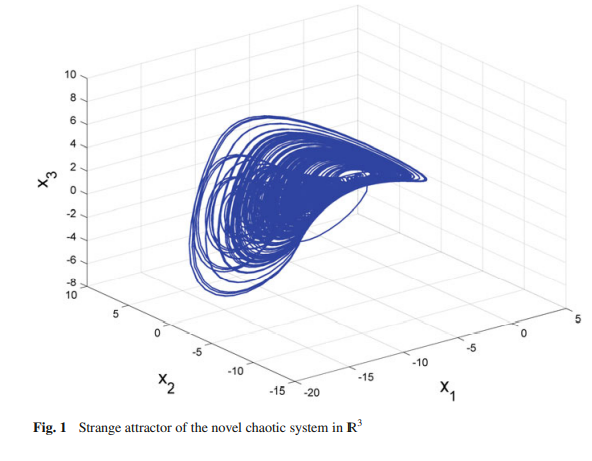
This research work describes an eight-term 3-D novel polynomial chaotic system consisting of three quadratic nonlinearities. First, this work presents the 3-D dynamics of the novel chaotic system and depicts the phase portraits of the system. Next, the qualitative properties of the novel chaotic system are discussed in detail. The novel chaotic system has four equilibrium points. We show that two equilibrium points are saddle points and the other equilibrium points are saddle-foci. The Lyapunov exponents of the novel chaotic system are obtained as L1 = 0.4715, L2 = 0 and L3 = -2.4728. The

An eight-term 3-D novel chaotic system with three quadratic nonlinearities, its adaptive feedback control and synchronization
This research work describes an eight-term 3-D novel polynomial chaotic system consisting of three quadratic nonlinearities. First, this work presents the 3-D dynamics of the novel chaotic system and depicts the phase portraits of the system. Next, the qualitative properties of the novel chaotic system are discussed in detail. The novel chaotic system has four equilibrium points. We show that two equilibrium points are saddle points and the other equilibrium points are saddle-foci. The Lyapunov exponents of the novel chaotic system are obtained as L1 = 0.4715, L2 = 0 and L3 = −2.4728. The
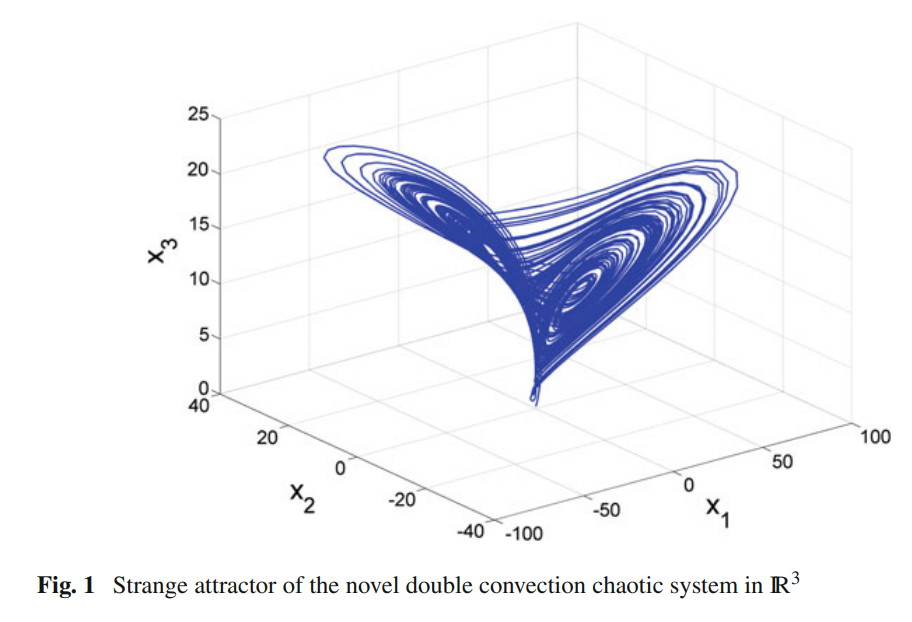
Adaptive control of a novel nonlinear double convection chaotic system
This research work describes a six-term novel nonlinear double convection chaotic system with two nonlinearities. First, this work presents the 3-D dynamics of the novel nonlinear double convection chaotic system and depicts the phase portraits of the system. Our novel nonlinear double convection chaotic system is obtained by modifying the dynamics of the Rucklidge chaotic system (1992). Next, the qualitative properties of the novel chaotic system are discussed in detail. The novel chaotic system has three equilibrium points. We show that the equilibrium point at the origin is a saddle point

Adaptive control of a novel nonlinear double convection chaotic system
This researchwork describes a six-term novel nonlinear double convection chaotic system with two nonlinearities. First, this work presents the 3-D dynamics of the novel nonlinear double convection chaotic system and depicts the phase portraits of the system. Our novel nonlinear double convection chaotic system is obtained by modifying the dynamics of the Rucklidge chaotic system (1992). Next, the qualitative properties of the novel chaotic system are discussed in detail. The novel chaotic system has three equilibrium points. We show that the equilibrium point at the origin is a saddle point
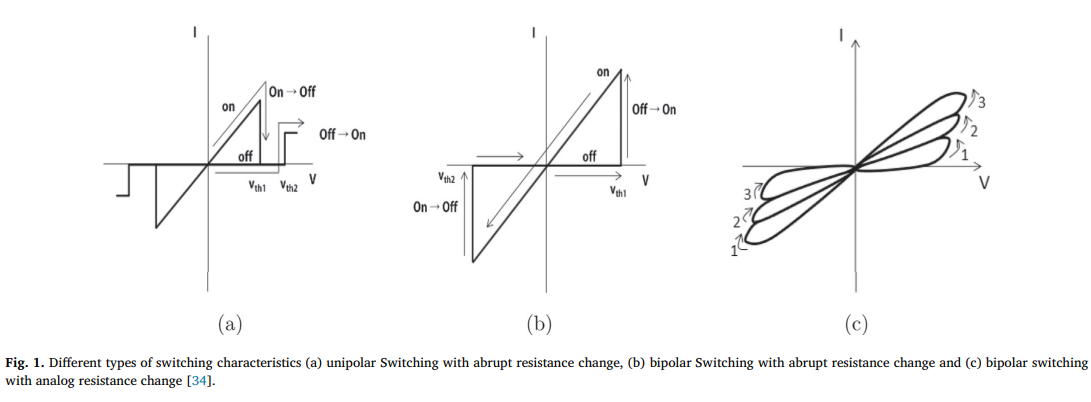
Design and analysis of 2T2M hybrid CMOS-Memristor based RRAM
In this paper, a Static Noise Margin (SNM) analysis for 2T2M RRAM cell is investigated. The proposed analysis is done using mathematical formulation and verified by SPICE simulations. The analysis is tested for both, write and read modes. Moreover, the analysis is applied to diverse types of RRAM cells, and a comparison between the performance of such cells is discussed. Additionally, the effect of the exponential memristor model on the memristor behaviour in terms of switching speed and the range of the memristor resistance are discussed in detail. The circuits design and simulations were
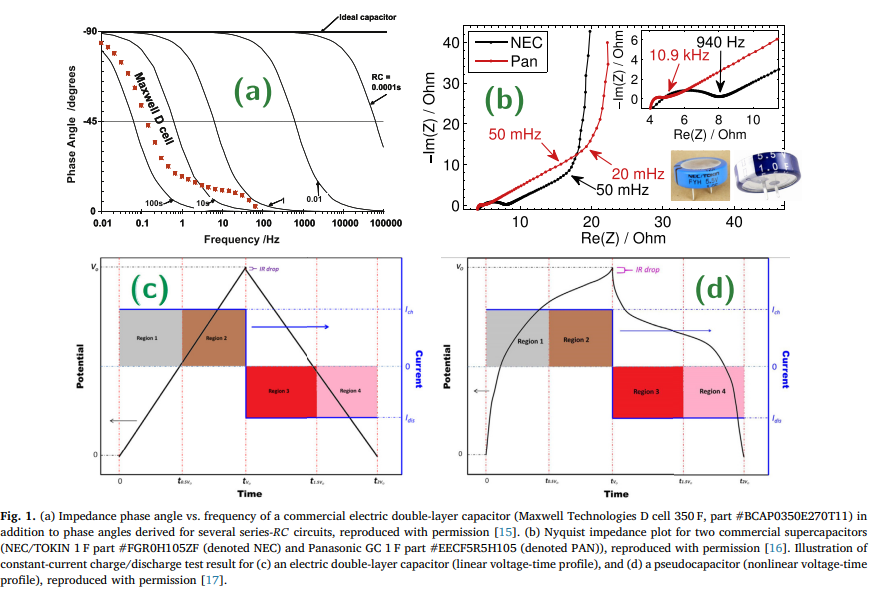
Review of fractional-order electrical characterization of supercapacitors
The tests and calculation of the key performance metrics of supercapacitors including capacitance, power and energy stored are commonly reported by the academia and the industry based on formulæ valid only for ideal capacitors. This is inconsistent with the fact that supercapacitors exhibit electrical behaviors that are different from those of ideal capacitors whether they are looked at in the time domain or in the frequency domain. This results inevitably in errors in their characterization, design and system integration. Based on recent literature, this review article is an attempt to
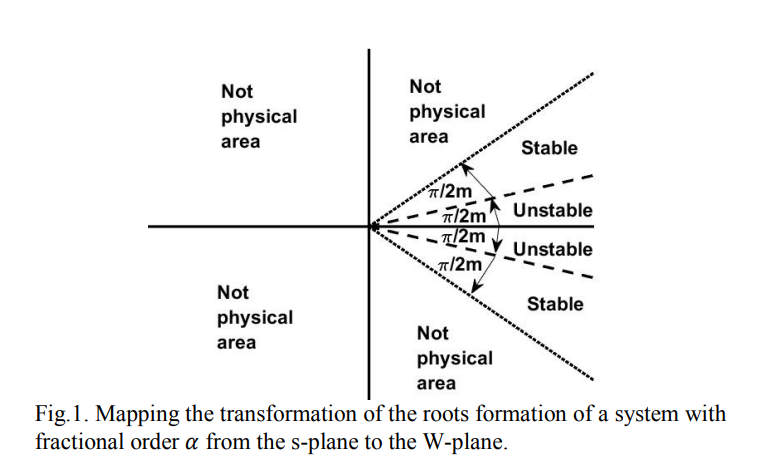
Generalization of third-order low pass filters to the fractional-order domain with experimental results
This paper is concerned with the generalization of continuous-time 3rd order low-pass filters to the fractional order domain. Three fractional-order capacitors of the same order α are to be considered in the process of filter design. A complete mathematical analysis of the 3rd order fractional low-pass filter is given with stability constraints taken into consideration. Finally, several low-pass active filter design examples are illustrated and discussed in the simulations section in terms of numerical MATLAB simulations, PSpice simulations and experimental results. © 2018 IEEE.
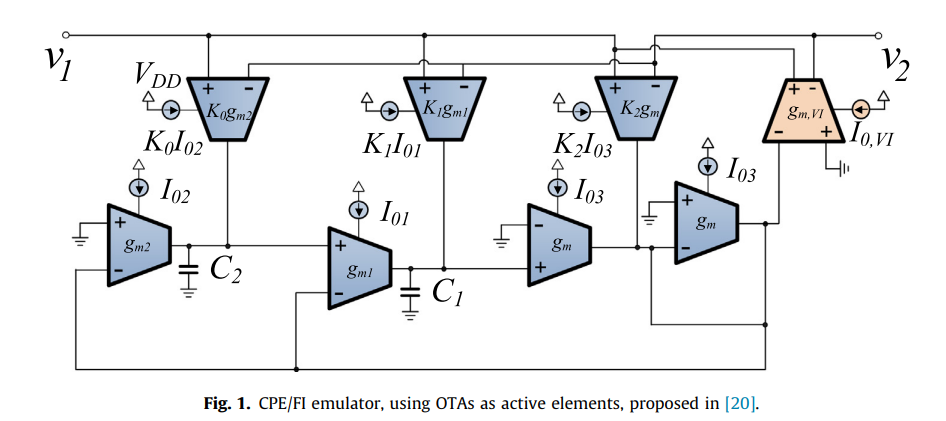
One-terminal electronically controlled fractional-order capacitor and inductor emulator
A novel topology for simultaneous emulation of fractional-order capacitors and inductors is presented in this paper. In particular, only one control terminal is used in order to select the type of the element as well as its fractional order. This is achieved through an appropriate fitting of the expressions of the intermediate bias currents, in such a way that, eventually, only the adjustment of one main bias current is required. The performance of the proposed topology is evaluated through post-layout simulations using Cadence and Austria Mikro Systeme (AMS) 0.35μm CMOS process. © 2019
Pagination
- Previous page ‹‹
- Page 27
- Next page ››