Breadcrumb
Fractional X-shape controllable multi-scroll attractor with parameter effect and FPGA automatic design tool software
This paper proposes a new fractional-order multi-scrolls chaotic system. More complex systems and flexible ranges of the chaotic behavior are obtained due to the extra parameters added by the fractional-order. The proposed system has novel complex chaotic behaviors. The effect of changing the system parameters on the system behavior is investigated and their bifurcation diagrams have been provided. The MLE for the proposed system in integer and fractional domain has been discussed. It shows that the proposed chaotic system is richer in the case of fractional-order. A novel FPGA design
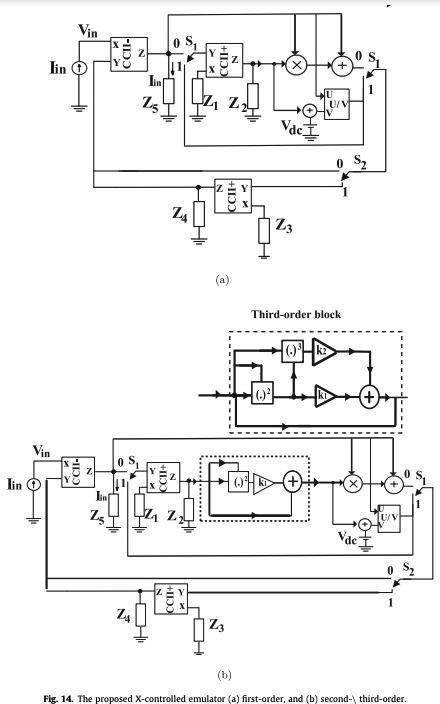
A general emulator for fractional-order memristive elements with multiple pinched points and application
In this paper, X-controlled universal fractional-order memelements (FOMEs) emulator is proposed. The emulation circuit is realized using second-generation current conveyor (CCII) and analog voltage multiplier (AVM)/divider block with two switches to control the type of memelements and emulator mode. The effect of the fractional-order capacitor (FOC) on the pinched hysteresis loop (HL) area and the range of frequency is discussed at different fractional-order α. Additionally, the higher-order meminductor, memristor, memcapacitor, and inverse memristor are discussed presenting multiple pinched
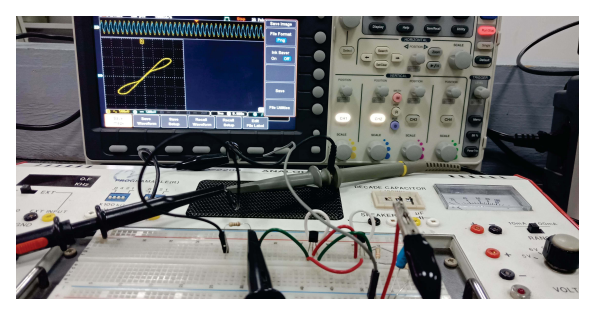
A Simple BJT Inverse Memristor Emulator and Its Application in Chaotic Oscillators
A generalized inverse memristor emulator is proposed based on two BJT transistors as a diode connected with a first order parallel RC filter. The mathematical model of the circuit is presented where the pinched hysteresis loops (PHLs) with different periodic stimuli are analyzed. The numerical, P-Spice simulations and experimental results are presented indicating that the introduced emulator is a simple voltage-controlled generalized inverse memristor. The results show that the PHLs area is increased with increasing the applied frequency. In addition, the proposed emulator is employed in a
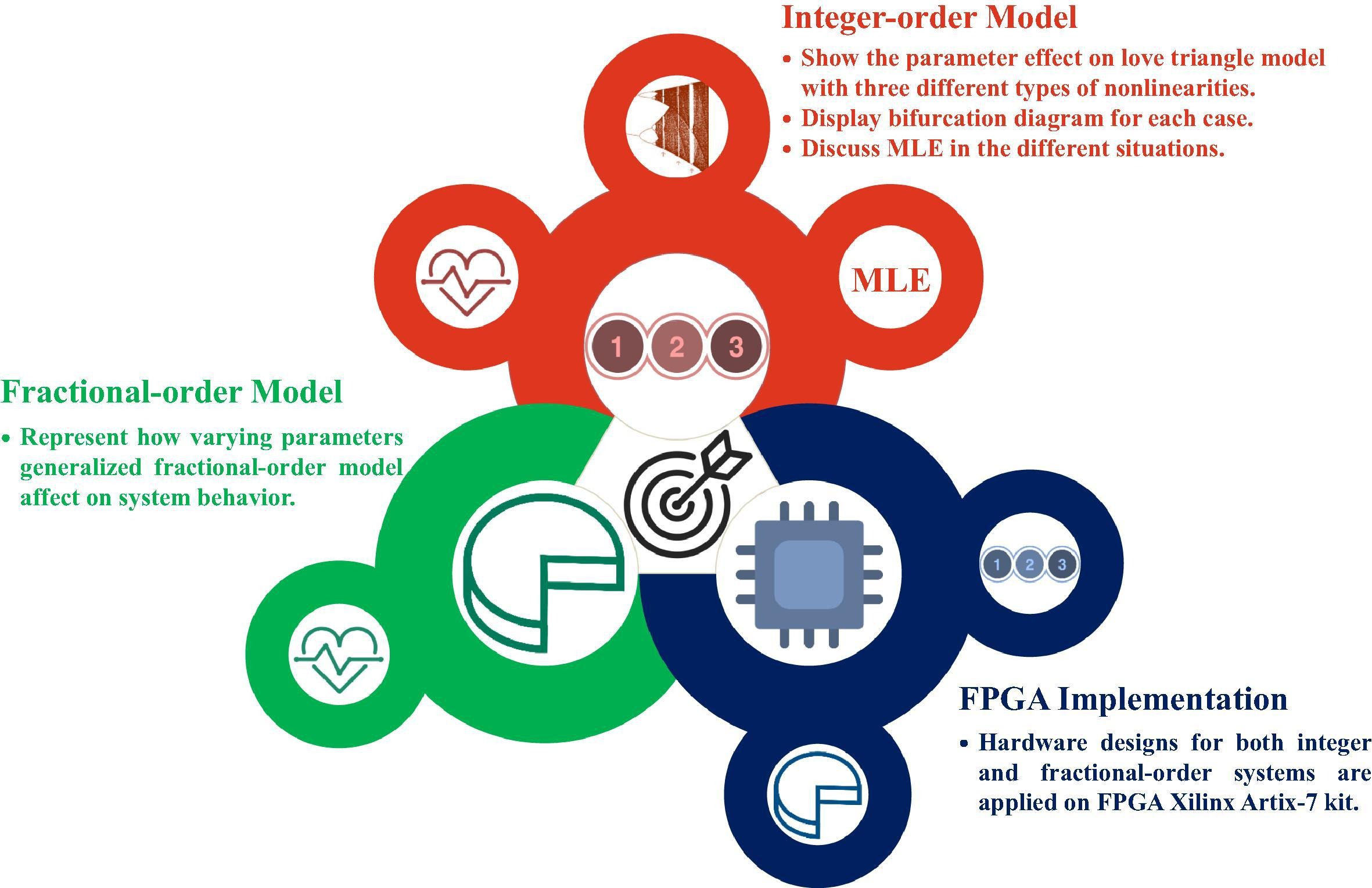
A study of the nonlinear dynamics of human behavior and its digital hardware implementation
This paper introduces an intensive discussion for the dynamical model of the love triangle in both integer and fractional-order domains. Three different types of nonlinearities soft, hard, and mixed between soft and hard, are used in this study. MATLAB numerical simulations for the different three categories are presented. Also, a discussion for how the kind of personalities affects the behavior of chaotic attractors is introduced. This paper suggests some explanations for the complex love relationships depending on the impact of memory (IoM) principle. Lyapunov exponents, Kaplan-Yorke
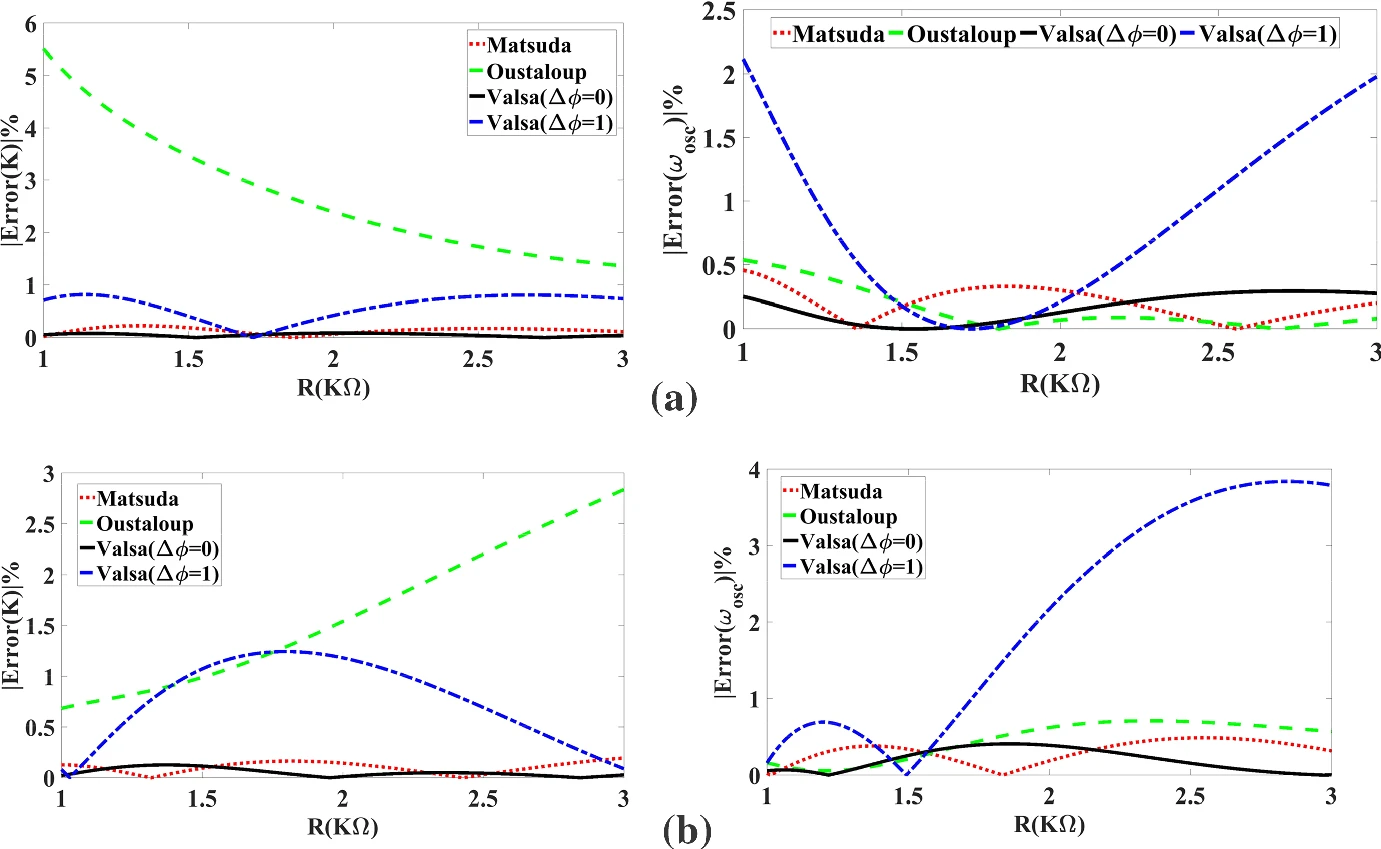
All Possible Topologies of the Fractional-Order Wien Oscillator Family Using Different Approximation Techniques
This paper introduces all the possible topologies of the Wien bridge oscillator family. This family has 72 topologies, 24 of them contain only RC or RL pairs, and the rest contain mixed pairs. The complete mathematical analysis of all twelve possible capacitive-based topologies is proposed in the fractional-order domain. The investigated circuits can be categorized into two groups, each with a similar characteristic equation. Three integer-order approximation techniques for the Laplacian operator sα are employed to solve and simulate the Wien bridge system. The studied approximations are those
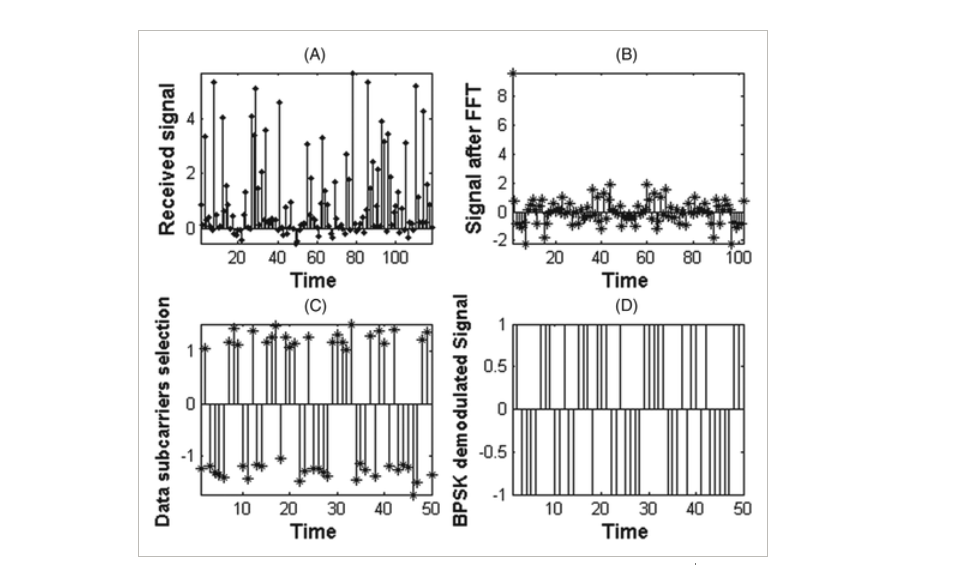
Odd clipping optical orthogonal frequency division multiplexing for VLC system
The Orthogonal Frequency Division Multiplexing (OFDM) has emerged as one of the promising techniques because of its robustness to multipath fading with high-speed data transmission. Classical bipolar OFDM cannot be used in intensity modulated with direct detection (IM/DD) optical communication systems, as visible light communication (VLC), so many optical modulation techniques as asymmetrical clipped optical OFDM (ACO-OFDM) and DC-Clipped OFDM (DCO-OFDM) have been investigated. In this paper, we introduce a novel optical modulation scheme that meets the optical communications requirements. The
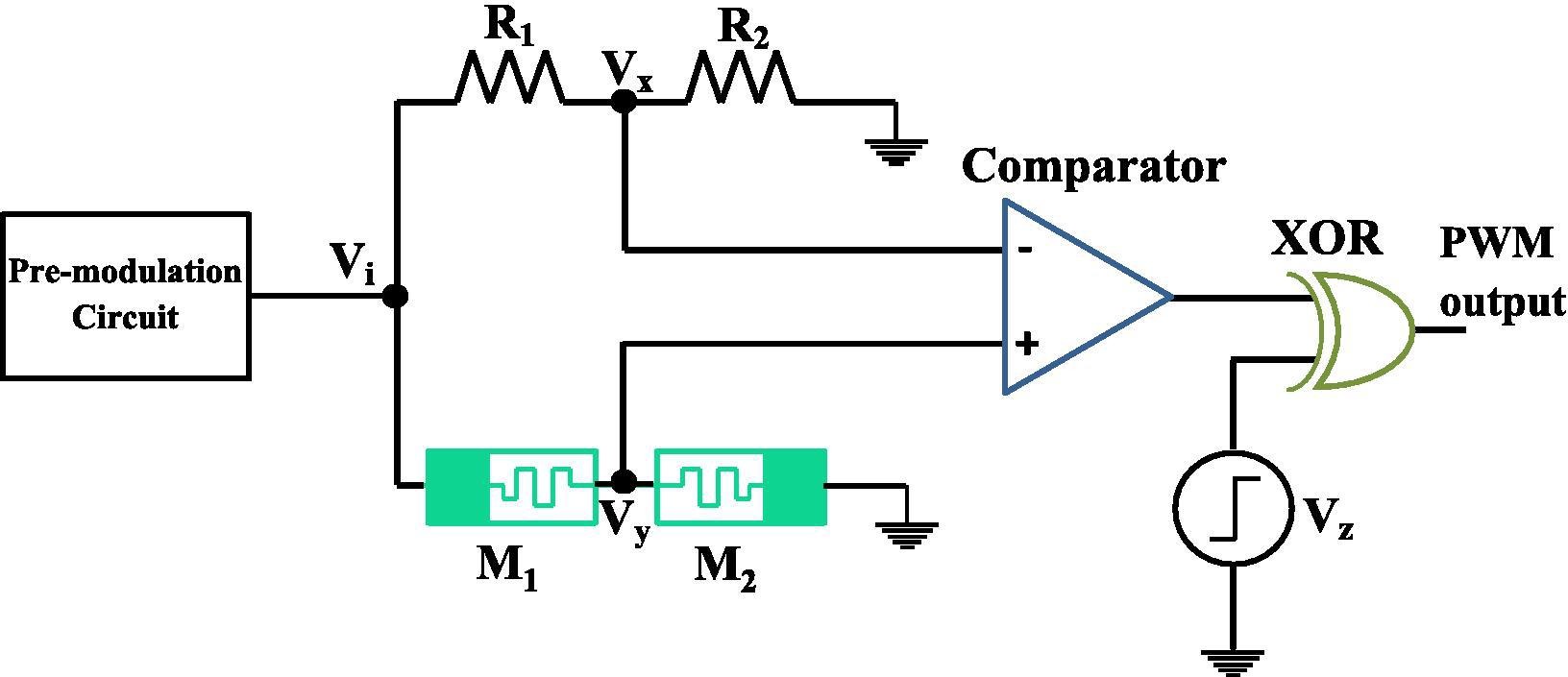
Center pulse width modulation implementation based on memristor
This paper introduces two new versions for memristor-based center pulse-width modulator (PWM) circuits. The proposed circuits use only one comparator which reduces the circuit complexity and power dissipation compared to a former work. The first design is based on two memristors and two resistors while the second design is based on four memristors. Theoretical analysis is provided, and the numerical solution is handled on MATLAB. Simulation is carried out on Cadence software, and the results follow the theoretical analysis. The experiment is implemented using commercial off-the-shelf
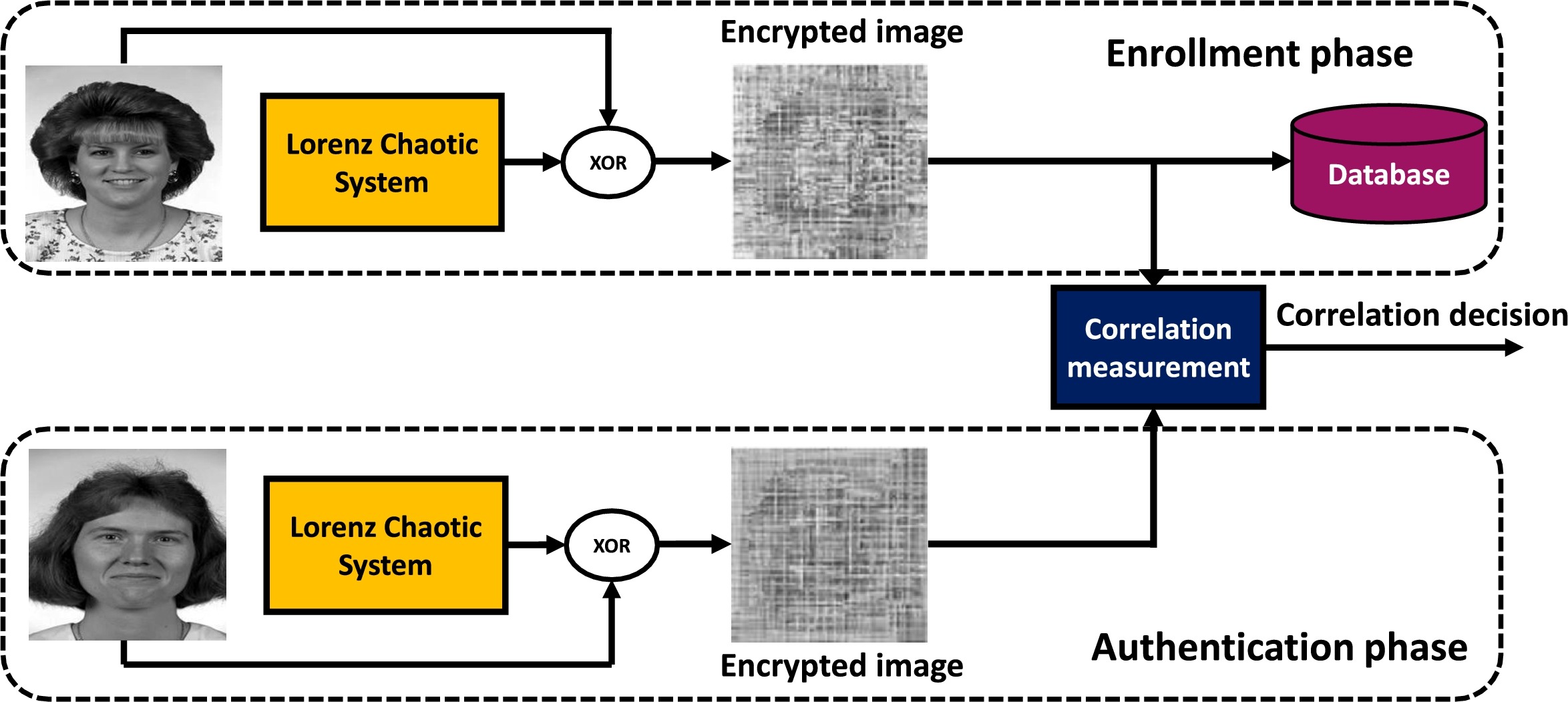
Cancellable face recognition based on fractional-order Lorenz chaotic system and Haar wavelet fusion
Cancellable biometrics is the art of generating distorted or encrypted templates of original biometric templates. The evolution of cancellable biometrics is attributed to the advanced hacking technologies that can capture the original stored biometrics from databases. One of the solutions for this problem is to store cancellable biometric templates in the database rather than the original ones. This paper presents a cancellable face recognition scheme that is based on face image encryption with Fractional-Order (FO) Lorenz chaotic system. The basic idea is to generate user-specific random keys
Two implementations of fractional-order relaxation oscillators
This work proposes general formulas for designing two different topologies of fractional-order relaxation oscillators. One topology contains an Operational Amplifier and the other one relies on an Operational Trans-Resistance Amplifier. The design procedure hinges on the general fractional-order natural and step responses of RC, which is proved in this work depending on Mittag Leffler function. The proposed topologies can be controlled to generate symmetrical and non-symmetrical square wave signals. They also benefit from the employment of fractional-order capacitors (FOCs), which makes it
Synchronization and FPGA realization of fractional-order Izhikevich neuron model
This paper generalizes the Izhikevich neuron model in the fractional-order domain for better modeling of neuron dynamics. Accurate and computationally efficient numerical techniques such as non-standard finite difference (NSFD) scheme is used to solve the neuron system in the fractional-order domain for different cases. Neuron synchronization plays an important role in the process of information exchange among coupled neurons. The general formula for the synchronization of different Izhikevich neurons is proposed. Also, the synchronization of two and three neurons are studied at different
Pagination
- Previous page ‹‹
- Page 14
- Next page ››