Breadcrumb
Digital Emulation of a Versatile Memristor with Speech Encryption Application
Memristor characteristics such as nonlinear dynamics, state retention and accumulation are useful for many applications. FPGA implementation of memristor-based systems and algorithms provides fast development and verification platform. In this work, we first propose a versatile digital memristor emulator that exhibits either continuous or discrete behaviors, similar to valence change memories (VCM) and the electrochemical metallization memories. Secondly, the proposed memristor emulator is used to design a chaotic generator circuit utilizing the memristor's nonlinearity. Finally, the chaotic
Fractional inverse generalized chaos synchronization between different dimensional systems
In this chapter, new control schemes to achieve inverse generalized synchronization (IGS) between fractional order chaotic (hyperchaotic) systems with different dimensions are presented. Specifically, given a fractional master system with dimension n and a fractional slave system with dimension m, the proposed approach enables each master system state to be synchronized with a functional relationship of slave system states. The method, based on the fractional Lyapunov approach and stability property of integer-order linear differential systems, presents some useful features: (i) it enables
Fractional inverse generalized chaos synchronization between different dimensional systems
In this chapter, new control schemes to achieve inverse generalized synchronization (IGS) between fractional order chaotic (hyperchaotic) systems with different dimensions are presented. Specifically, given a fractional master system with dimension n and a fractional slave system with dimension m, the proposed approach enables each master system state to be synchronized with a functional relationship of slave system states. The method, based on the fractional Lyapunov approach and stability property of integer-order linear differential systems, presents some useful features: (i) it enables
Fractional-order oscillators
Fractional-order calculus is the branch of mathematics which deals with non-integerorder differentiation and integration. Fractional calculus has recently found its way to engineering applications; particularly electronic circuits with promising results showing the feasibility of fabricating fractional-order capacitors on silicon. Fractionalorder capacitors are lossy non-deal capacitors with an impedance given by Zc = (1/jωC)α, where C is the pseudo-capacitance and α is its order (0
Fractional order butterworth filter: Active and passive realizations
This paper presents a general procedure to obtain Butterworth filter specifications in the fractional-order domain where an infinite number of relationships could be obtained due to the extra independent fractional-order parameters which increase the filter degrees-of-freedom. The necessary and sufficient condition for achieving fractional-order Butterworth filter with a specific cutoff frequency is derived as a function of the orders in addition to the transfer function parameters. The effect of equal-orders on the filter bandwidth is discussed showing how the integer-order case is considered
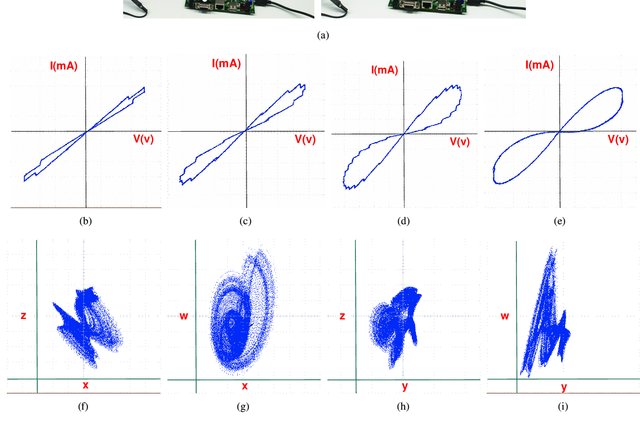
A three-dimensional no-equilibrium chaotic system: Analysis, synchronization and its fractional order form
Recently, a new classification of nonlinear dynamics has been introduced by Leonov and Kuznetsov, in which two kinds of attractors are concentrated, i.e. self-excited and hidden ones. Self-excited attractor has a basin of attraction excited from unstable equilibria. So, from that point of view, most known systems, like Lorenz's system, Rössler's system, Chen's system, or Sprott's system, belong to chaotic systems with self-excited attractors. In contrast, a few unusual systems such as those with a line equilibrium, with stable equilibria, or without equilibrium, are classified into chaotic

A three-dimensional no-equilibrium chaotic system: Analysis, synchronization and its fractional order form
Recently, a new classification of nonlinear dynamics has been introduced by Leonov and Kuznetsov, in which two kinds of attractors are concentrated, i.e. self-excited and hidden ones. Self-excited attractor has a basin of attraction excited from unstable equilibria. So, from that point of view, most known systems, like Lorenz’s system, Rössler’s system, Chen’s system, or Sprott’s system, belong to chaotic systems with self-excited attractors. In contrast, a few unusual systems such as those with a line equilibrium, with stable equilibria, or without equilibrium, are classified into chaotic
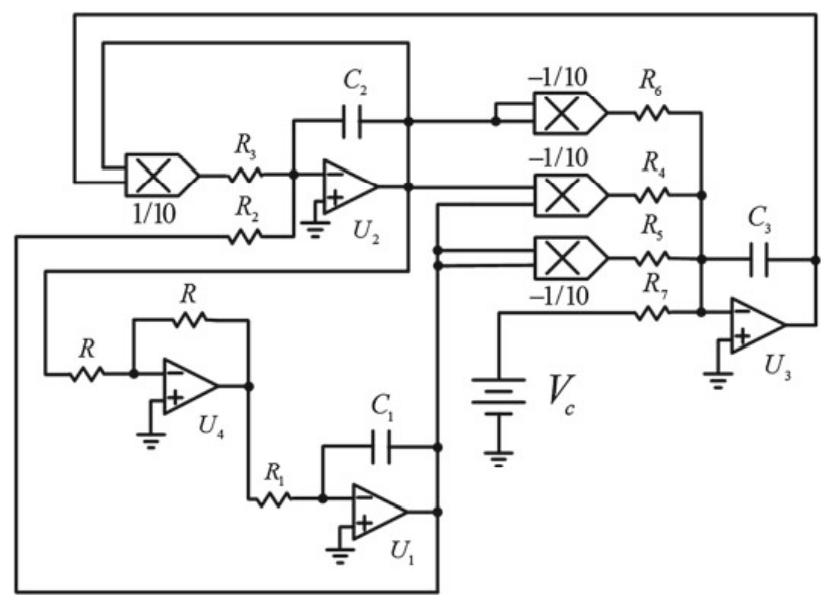
Band-Pass Filter and Relaxation Oscillator using Electric Double-Layer Capacitor
Supercapacitors are electrochemical devices that can store and restore electrical energy and are mostly used for powering dc or close-to-dc applications. As such they have not been explored enough for non-dc circuits. In this study, we implement a band-pass for frequency selectivity purposes and a relaxation oscillator for timing applications using a solid-state carbon electric double-layer capacitor. The expected behavior was observed for both circuits in the sub-Hertz and tens of Hertz frequency ranges. This confirms the possibility of using the frequency-dependent capacitive behavior of
Single transistor fractional-order filter using a multi-walled carbon nanotube device
A low-pass fractional-order filter topology based on a single metal oxide semiconductor transistor is presented in this Letter. The filter is realized using a fractional-order capacitor fabricated using multi-walled carbon nanotubes. The electronic tuning capability of the filter’s frequency characteristics is achieved through a biasing current source. Experimental results are presented and compared with the theory. © 2019, Springer Science+Business Media, LLC, part of Springer Nature.
Fractional order Chebyshev-like low-pass filters based on integer order poles
Chebyshev filter is one of the most commonly used prototype filters that approximate the ideal magnitude response. In this paper, a simple and fast approach to create fractional order Chebyshev-like filter using its integer order poles is discussed. The transfer functions for the fractional filters are developed using the integer order poles from the traditional filter. This approach makes this work the first to generate fractional order transfer functions knowing their poles. The magnitude, phase, step responses, and group delay are simulated for different fractional orders showing their
Pagination
- Previous page ‹‹
- Page 33
- Next page ››