Breadcrumb
Estimating phase error using a Hilbert transform-based time-domain technique
Measuring phase noise in oscillators is crucial in communication systems, vibration analysis, and frequency synthesizers. Traditionally, this measurement is done in frequency domain by estimating the ratio of the power density at an offset frequency from the carrier to the power of the carrier signal. This approach is hardware intensive and dependent on the the offset frequency, for which there exists no standard. Here, we propose an alternative method to quantify phase noise but in the time domain in the form of a root-mean-square true phase angle deviation. This is done using a self-created
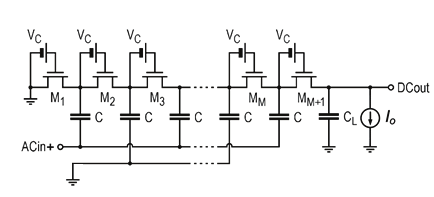
A mathematical model of an ideally threshold compensated rectifier for RF energy harvesting
This paper introduces a mathematical model of an ideally threshold compensated rectifier for RF energy harvesting. The ideally compensation arrangement has been exploited to improve the rectifier's performance and overcome the limitation of rectifier's sensitivity which mainly depends on the threshold voltage of the rectifying devices (transistors). The model considers the conduction angle and the reverse current in deriving closed form analytical expressions for output dc voltage and efficiency. Using a 65-nm low leakage CMOS process with low-threshold transistors, 900-MHz multi-stages
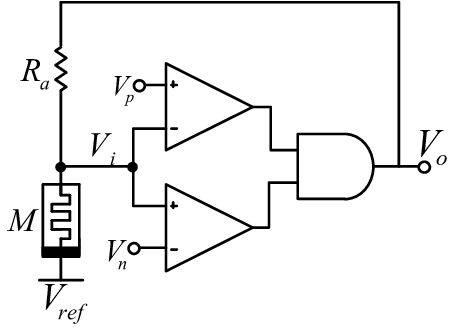
Effect of boundary on controlled memristor-based oscillator
Recently, the applications of memristors have spread into many fields and especially in the circuit theory. Many models have been proposed for the HP-memristor based on the window functions. In this paper, we introduce a complete mathematical analysis of the controlled reactance-less oscillator for two different window functions of Joglekar's model (linear and nonlinear dopant drift) to discuss the effect of changing the window function on the oscillator's behavior. The generalized necessary and sufficient conditions based on the circuit elements and control voltages for both the linear and
Power dissipation of memristor-based relaxation oscillators
Recently, many reactance-less memristive relaxation oscillators were introduced, where the charging and discharging processes depend on memristors. In this paper, we investigate the power dissipation in different memristor based relaxation oscillators. General expressions for these memristive circuits as well as the power dissipation formulas for three different topologies are derived analytically. In addition, general expressions for the maximum and minimum power dissipation are calculated. Finally, the calculated expressions are verified using PSPICE simulations showing very good matching.
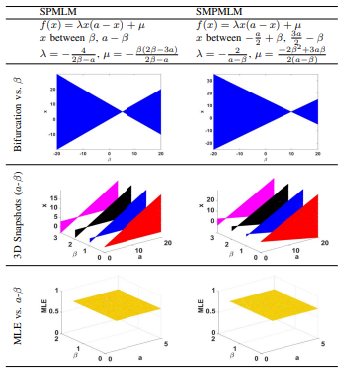
Speech encryption using generalized modified chaotic logistic and tent maps
This paper presents a speech encryption application, which utilizes several proposed generalized modified discrete chaotic maps based on the logistic and tent maps for pseudo-random number generation. The generalization scales the output range and the key space. The modification controls the bounds on the output range through a parameter such that chaotic output exists for almost all values of the parameter. Consequently, the modified maps do not suffer from the inherited problems of conventional chaotic generators such as islands of stability and drifting from chaos due to dynamical
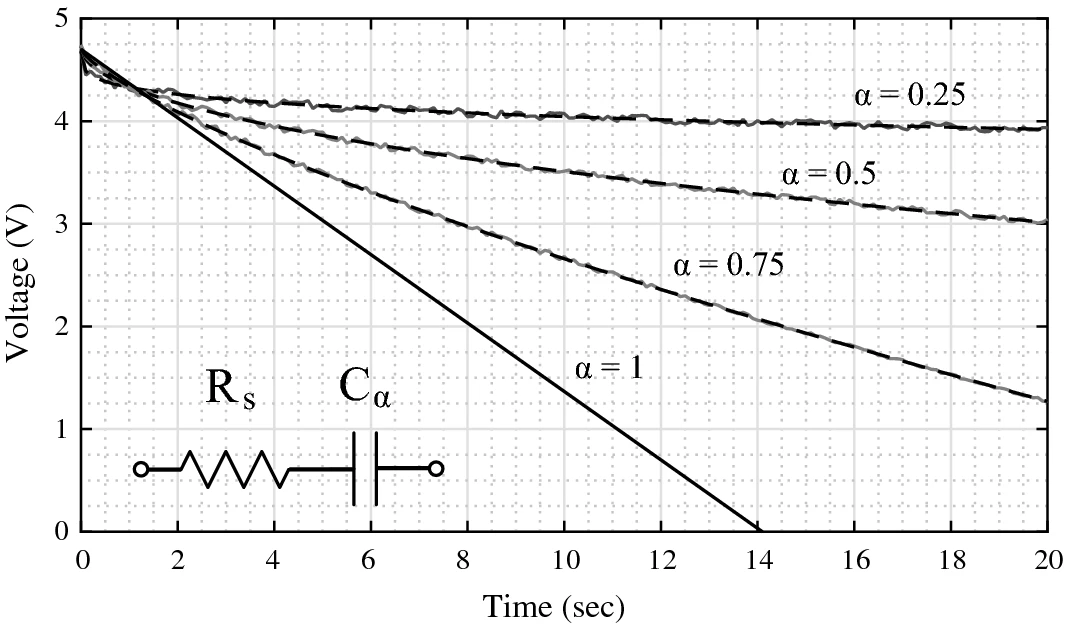
Rates and Effects of Local Minima on Fractional-Order Circuit Model Parameters Extracted from Supercapacitor Discharging Using Least Squares Optimization
Optimization routines are widely used to numerically determine a set of model parameters that best fit collected experimental data. One recent application of these methods is to extract the fractional-order circuit model parameters that accurately characterize the transient behavior of discharging supercapacitors. However, the variability that these methods introduce to the extracted model parameters must be understood to determine if changes in model parameters are artifacts of the optimization routine or are representative of physical changes in the device under study. In this work, the
Fractional-order Fitzhugh-Nagumo and Izhikevich neuron models
This paper studies the famous Fitzhugh-Nagumo and Izhikevich neuron models in the fractional-order domain. Generalization of the integer models into the fractional-order domain providing a wider scope understanding of the neuron systems. The fractional Fitzhugh-Nagumo circuit model and the state space equations are introduced. Different fractional orders are studied as an example. Numerical solutions of the systems are given using non-standard finite difference scheme together with Grunwald-Letnikov discretization technique which is computationally efficient and accurate. The two models are
Elmore delay in the fractional order domain
Interconnect design has recently become one of the important factors that affect the circuit delay and performance especially in the deep submicron technology. The modelling of interconnects is typically based on using Elmore definitions of the delay time and rise time. So, a general formula for Elmore delay time and rise time in the fractional order domain are presented in this work. It is found from the new formulas of the delay time and rise time that these timing values could be controlled or tuned by the fractional orders. Hence, the fractional order can compensate for the components
Electronically tunable fractional-order highpass filter for phantom electroencephalographic system model implementation
The fractional-order model of a phantom electroencephalographic system, at various distances between electrodes, is realized using appropriate decomposition of the rational transfer functions which approximate the highpass filters that describe its dynamics. The main offered benefits, in comparison to the corresponding straightforward implementations of the rational transfer functions, are the capability of monolithic implementation due the minimization of the maximum value of the required capacitances and, also, the reduced power consumption. The performance of the presented filter topologies
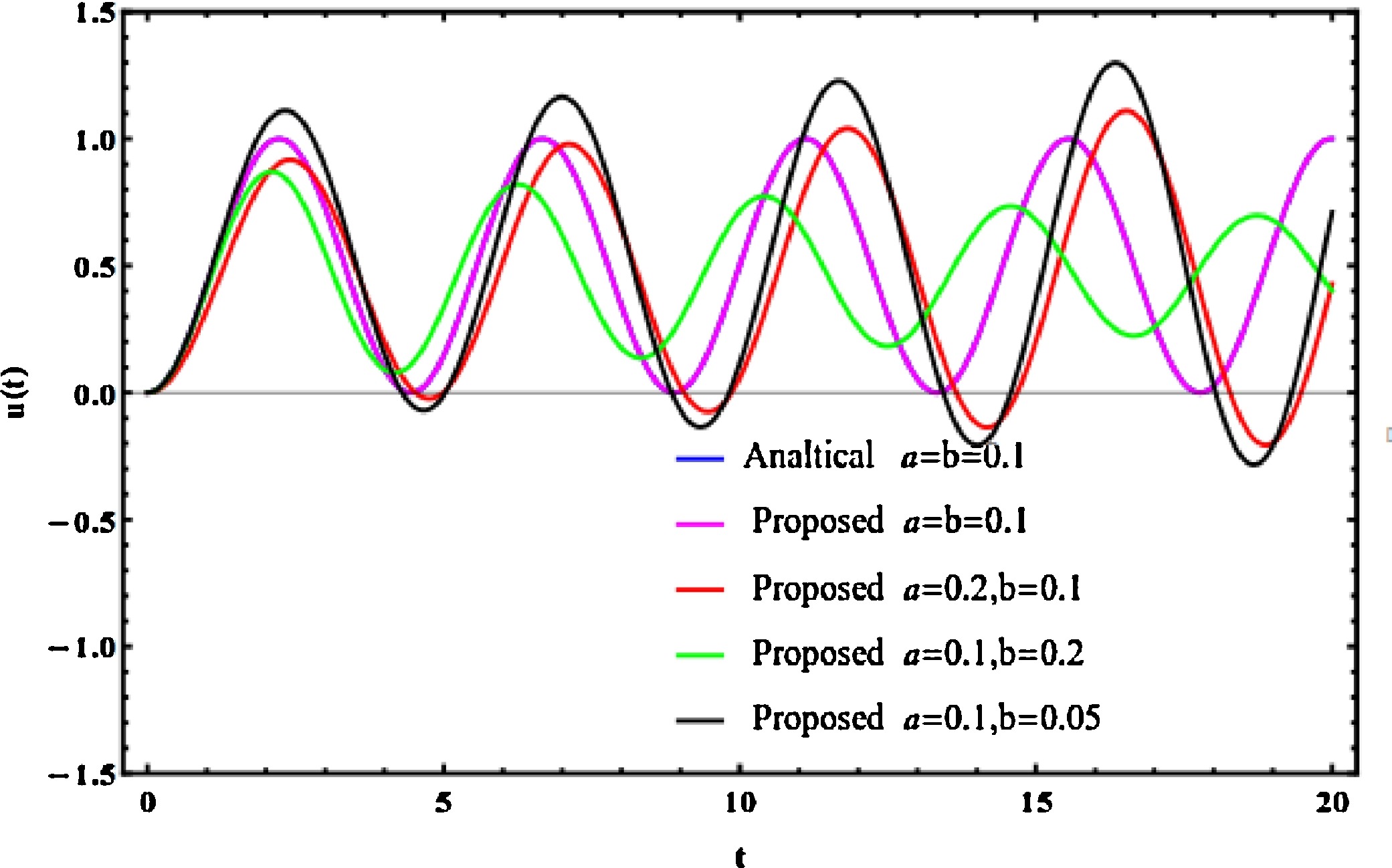
Modified methods for solving two classes of distributed order linear fractional differential equations
This paper introduces two methods for the numerical solution of distributed order linear fractional differential equations. The first method focuses on initial value problems (IVPs) and based on the αth Caputo fractional definition with the shifted Chebyshev operational matrix of fractional integration. By applying this method, the IVPs are converted into simple linear differential equations which can be easily handled. The other method focuses on boundary value problems (BVPs) based on Picard's method frame. This method is based on iterative formula contains an auxiliary parameter which
Pagination
- Previous page ‹‹
- Page 37
- Next page ››