Breadcrumb
Multiple Pinch-Off Points in Memristive Equations: Analysis and Experiments
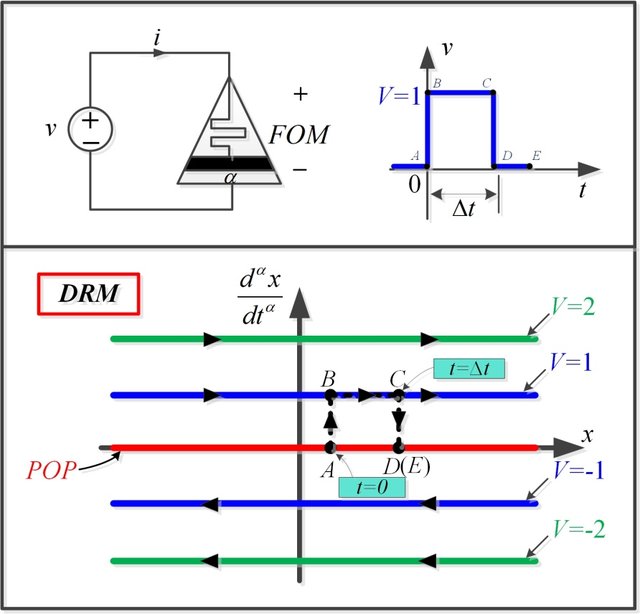
Pinched hysteresis behavior is considered evidence of the existence of the memristive element. Recently, the multi-lobes (more than two) behavior has been discovered in some memristive devices. In this paper, a fractional-order flux/voltage-controlled memristive model is introduced that is able to develop multiple symmetric and asymmetric pinch-off points. Generalized closed-form expressions for the necessary conditions of multiple pinch-off points existence are derived in addition to the coordinates of the pinch-off points in the I-V plane. Closed-form expressions for the minimum and maximum achievable resistances are derived. Moreover, different illustrative numerical examples have been given verifying the calculated expressions. Finally, in order to verify the multiple pinch-off behaviors, the proposed emulator circuit is introduced, analyzed, and verified using both SPICE simulations and experiments to validate the proposed models. Different fractional-order cases have been experimentally tested using different excitation signals such as sinusoidal, square, and triangular signals showing the circuit-ability to generate the multiple pinch-off points. © 2019 IEEE.